Click here👆to get an answer to your question ️ Factorise x(x y)^3 3x^2y(x y) How do you factor this polynomial completely?Click here👆to get an answer to your question ️ Factorise (x y)^3 (y z)^3 (z x)^3
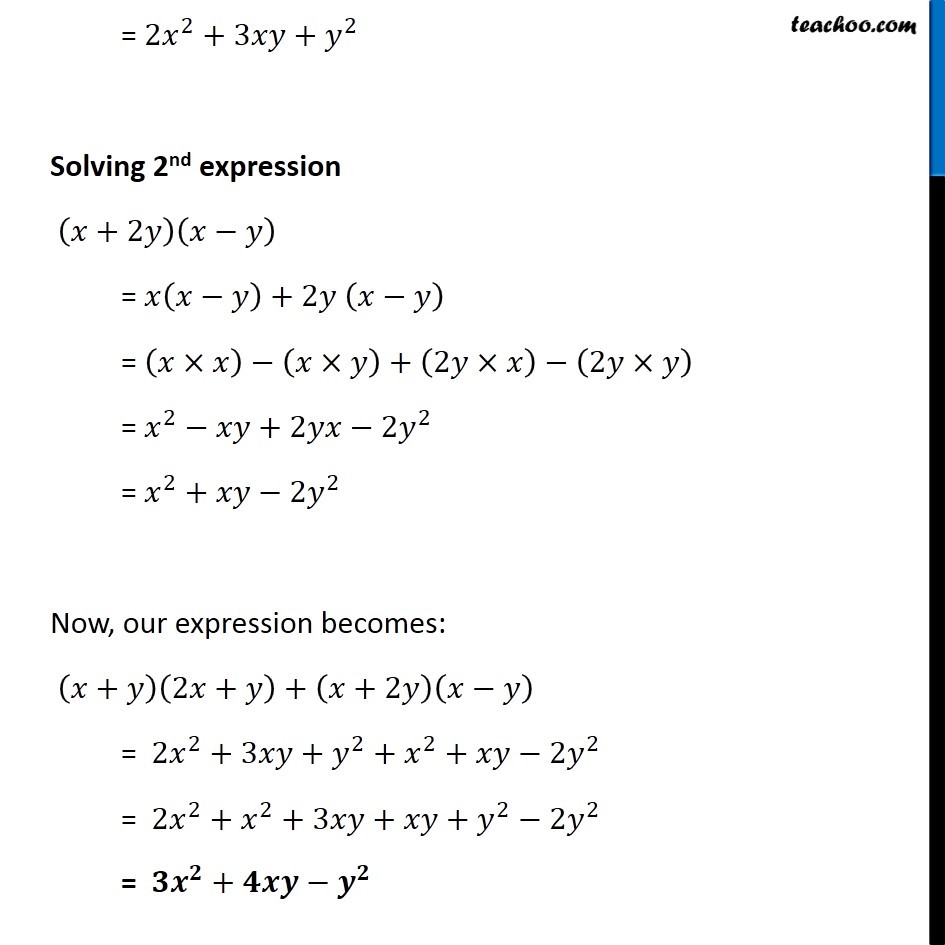
Ex 9 4 3 V Simplify X Y 2x Y X 2y X Y Class 8
Factorize x(x-y)^(3)+3x^(2)y(x-y)
Factorize x(x-y)^(3)+3x^(2)y(x-y)-(a) x 2 y 2 2xy (b) x 2 y 2 – xy (c) xy 2 (d) 3xy polynomialsAlgebra Polynomials and Factoring Special Products of Polynomials 1 Answer Don't Memorise We can write this expression as #x^3(2y)^3# The formula for factorizing the Difference of two Cubes is #a^3−b^3=(a−b)(a^2abb^2)# In #x^3(2y)^3#, #a=x# #b=2y# #x^3(2y)^3 #
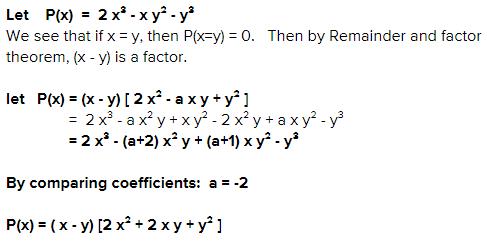



Factorise 2x 3 Xy 2 Y 3 Edurev Class 9 Question
Which of the following is a factor of (x y) 3 – (x 3 y 3 )?Are you asking about this specific division, or for arbitrary polynomial divisions?Maths Factorise x33x2y3xy2y3−125=0 Answer x33x2y3xy2y3−125=0 ⇒(x33x2y3xy2y3)=125 ⇒x33xy(xy)y3=125 Using the identity,
At first glance math(0,1)/math and math(1,0)/math are solutions If it has a nice linear solution, fitting this then that would be mathy= x1/math, letsWe think you wrote (x^3y^3)dx3xy^2dy=0 This deals with factoring binomials as the sum or difference of cubesFactor out the Greatest Common Factor (GCF), '4dxy' 4dxy(y 2 1x 2) = 0 Factor a difference between two squares 4dxy((y x)(y 1x)) = 0 Ignore the factor 4 Subproblem 1 Set the factor 'dxy' equal to zero and attempt to solve Simplifying dxy = 0 Solving dxy = 0 Move all terms containing d to the left, all other terms to the right
Equations Tiger Algebra gives you not only the answers, but also the complete step by step method for solving your equations x^39y^33xy(xy) so that you understand betterStart with a number 3 since it's common to all three terms and we factor 3 out Step 2 Now look at the xterms with the highest exponent in x that is common to all three terms In this case, it's simply x and we factor x out Step 3 Now look at the yterms and we observe that is common to all three terms and we factor out Step 4 ANSWERXy=3;3x2y=4 Simple and best practice solution for xy=3;3x2y=4 Check how easy it is, to solve this system of equations and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the system of equations solver your own




Answers



Www Tau Ac Il Levant Ode Solution 6 Pdf
Step 3 Checking for a perfect cube 31 Factoring x33x2y3xy2y3 x33x2y3xy2y3 is a perfect cube which means it is the cube of another polynomial In our case, the cubic root of x33x2y3xy2y3 is xy Factorization is (xy)3Steps for Solving Linear Equation 3x2y=4 3 x 2 y = 4 Subtract 2y from both sides Subtract 2 y from both sides 3x=42y 3 x = 4 − 2 y Divide both sides by 3 Divide both sides by 3 Click here 👆 to get an answer to your question ️ factor x(xy)^3 3x^2y(xy) Mingyurpaldon7263 Mingyurpaldon7263 Math Secondary School answered Factor x(xy)^3 3x^2y(xy) 1 See answer Mingyurpaldon7263 is waiting for your help Add your answer and earn points
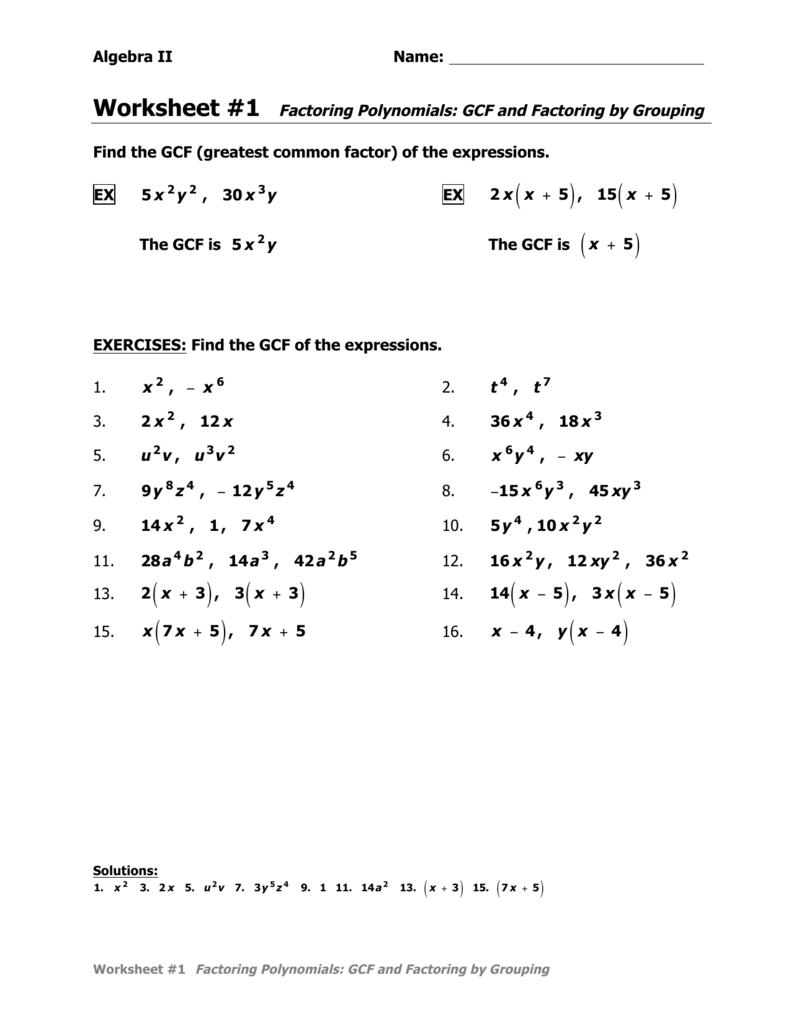



Hw 4 Polynomial Operations Doc




Factoring Two Variable Quadratics Rearranging Video Khan Academy
Algebra Factor (xy)^3 (xy)^3 (x y)3 − (x − y)3 ( x y) 3 ( x y) 3 Since both terms are perfect cubes, factor using the difference of cubes formula, a3 −b3 = (a−b)(a2 abb2) a 3 b 3 = ( a b) ( a 2 a b b 2) where a = xy a = x y and b = x− y b = x y Take out the common factor of #x# first #y = x^3 3x^2 10x# #y = x(x^23x10)# To factorise the quadratic trinomial, find factors of #10# which subtract to give #3# #15xx2 = 10 and 52 =3# so these are the factors we need Their signs must be different, but there must be more negativesSolve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more




The Factors Of X 3 X 2y X Y 2 Y 3 Are A X Y X 2 X Y Y 2 B X Y X 2 X Y Y 2 C Youtube



1
3x26xy3y2 Final result 3 • (x y)2 Step by step solution Step 1 Equation at the end of step 1 ( (3 • (x2)) 6xy) 3y2 Step 2 Equation at the end of step 2 (3x2 6xy) 3y2 Step Factor out 3 Consider x^ {2}y2xy3xy^ {2} Factor out xy Rewrite the complete factored expressionTo ask Unlimited Maths doubts download Doubtnut from https//googl/9WZjCW Factorise `x^32x^2x2`Find dy/dx x^3y^3=3x^2y3xy^2 x3 y3 = 3x2y 3xy2 x 3 − y 3 = 3 x 2 y − 3 x y 2 Differentiate both sides of the equation d dx (x3 y3) = d dx(3x2y 3xy2) d d x ( x 3 − y 3) = d d x ( 3 x 2 y − 3 x y 2) Differentiate the left side of the equation Tap for more steps Differentiate
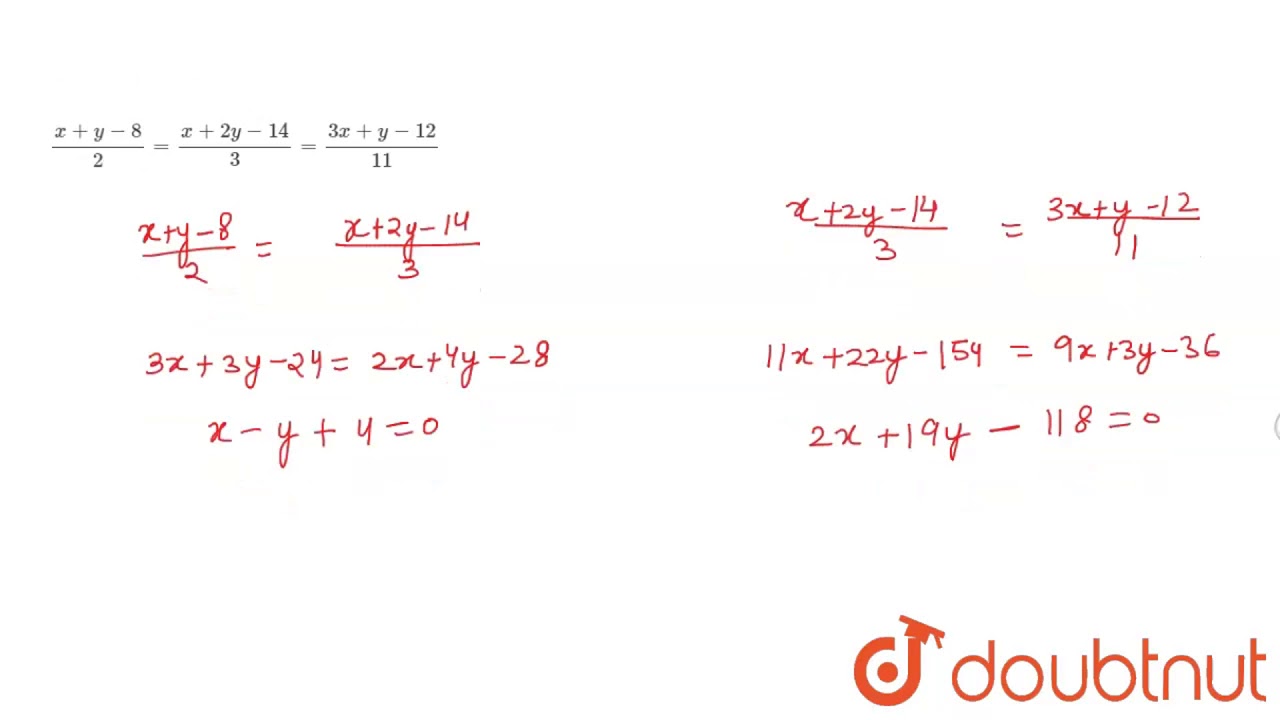



X Y 8 2 X 2y 14 3 3x Y 12 11 Youtube




Evaluate Please X 2 Y 2 3 Y 2 Z 2 3 Z 2 X 2 3 Divided By X Y 3 Y Z 3 Z X 3 Brainly In
Avail 25% off on study pack Avail OfferX^33x^2y3xy^2y^3 ———————————— X^3y^3 Get the answers you need, now!To make x and 3x equal, multiply all terms on each side of the first equation by 3 and all terms on each side of the second by 1 3x3y=9,3x2y4=0 Simplify 3x3x3y2y4=9 Subtract 3x2y4=0 from 3x3y=9 by subtracting like terms on each side of the equal sign 3y2y4=9




Simplify X 2 Y 2 3 Y 2 Z 2 3 Z 2 X 2 3 X Y 3 Y Z 3 Z X 3 Novocom Top




Factorize 2x 3 Xy 2 Y 3 Mathematics Topperlearning Com Uztky8oo
PreAlgebra Factor by Grouping x^33x^2y3xy^2y^3 x3 3x2y 3xy2 y3 x 3 3 x 2 y 3 x y 2 y 3 The polynomial cannot be factored using the grouping method Try a different method, or if you aren't sure, choose Factor The polynomial cannot be factored using the grouping method1 Log in Join now 1 Log in Join now Ask your question 35aryan Mathematics High School 5 pts Answered X^33x^2y3xy^2y^3Click here👆to get an answer to your question ️ If x y and y 2x are two factor of the expression x^3 3x^2y lambda xy^2 mu y^3 , then
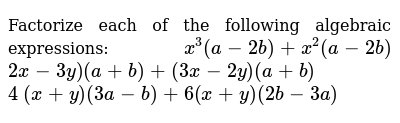



Factorize X X Y 3 3x 2y X Y
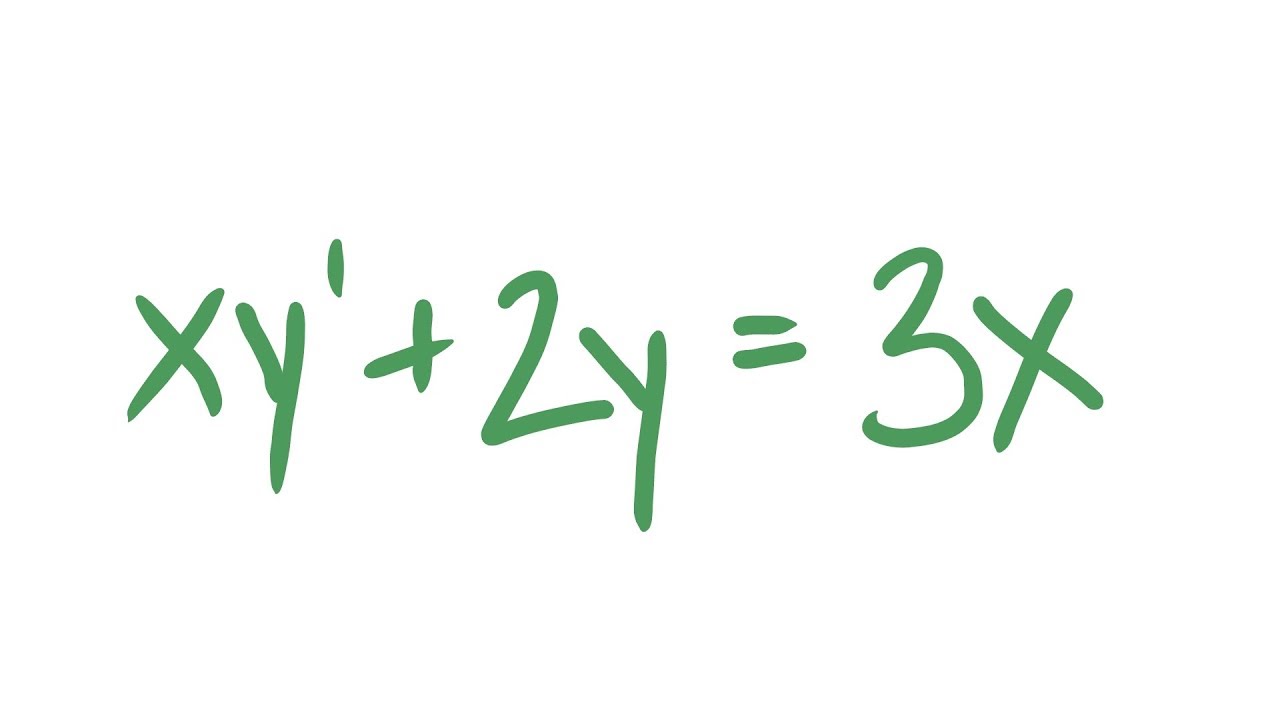



Exact Differential Equations With Integrating Factor Example 1 Xy 2y 3x Old Videos Youtube
Simplifying (2x 3 1xy 2 2y 3) * dx 1(x 2 y 2x) * dy = 0 Reorder the terms Factor out the Greatest Common Factor (GCF), 'dx' dx(3 4y 2xy 2 2x 3) = 0 Subproblem 1 Set the factor 'dx' equal to zero and attempt to solve Simplifying dx = 0 Solving dx = 0 Move all terms containing d to the left, all other terms to theClick here👆to get an answer to your question ️ factorise x^3 3x^2y 3xy^2 y^3 8For this specific example, Mr Paxson's answer is succinct, absolutely correct and cannot be improved If you recognise the pattern of binomial coefficients with ma




Facorize X X Y 3 3x 2y X Y




If X Y And 3x Y Are Two Factors Of The Expression Lambdax 3 Mux
We think you wrote x^33x^2y3xy^2y^3/x^33xy^22y^3 This deals with reducing fractions to their lowest termsSolve ( x 2 y − 2 x y 2) d x − ( x 3 − 3 x 2 y) d y = 0 written 34 years ago by smitapn612 ♦ 90 modified 15 months ago by sanketshingote ♦ 570 ADD COMMENT 1 Answer 1 658 views written 34 years ago by smitapn612 ♦ 90 modified 33 years ago by awariswati1 ♦ 770 Factorise x(xy)^33x^2y(xy) st is waiting for your help Add your answer and earn points




X 2 2y 3 1 And X Y 3 3 Find X And Y Values Using Elimination And Substitution Method Youtube




Factorize X X Y 2 3x2y X Y Brainly In
Equations Tiger Algebra gives you not only the answers, but also the complete step by step method for solving your equations x(xy)^33x^2y(xy) so that you understand betterGet stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!Factor x^3y^3 x3 − y3 x 3 y 3 Since both terms are perfect cubes, factor using the difference of cubes formula, a3 −b3 = (a−b)(a2 abb2) a 3 b 3 = ( a b) ( a 2 a b b 2) where a = x a = x and b = y b = y (x−y)(x2 xyy2) ( x y) ( x 2 x y y 2)
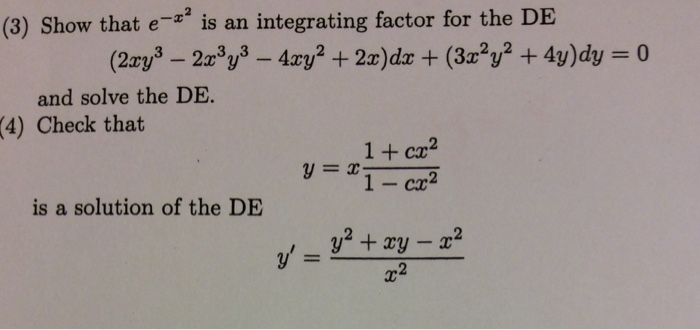



Show That E X 2 Is An Integrating Factor For The De Chegg Com




Simplify X Y 3 X Y 3 6y X 2 Y 2
Stack Exchange network consists of 177 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack ExchangeFactor (xy)^23(yx)^3 Rewrite as Expand using the FOIL Method Tap for more steps Apply the distributive property Apply the distributive property Apply the distributive property Simplify and combine like terms Tap for more steps Simplify each term Tap for more stepsFactor the trinomial x^2 2x 3




Class 9 Polynomial 2 Coordinate Geometry Linear Equation In Two Variables Euclid S Geometry Lines And Angles Notes
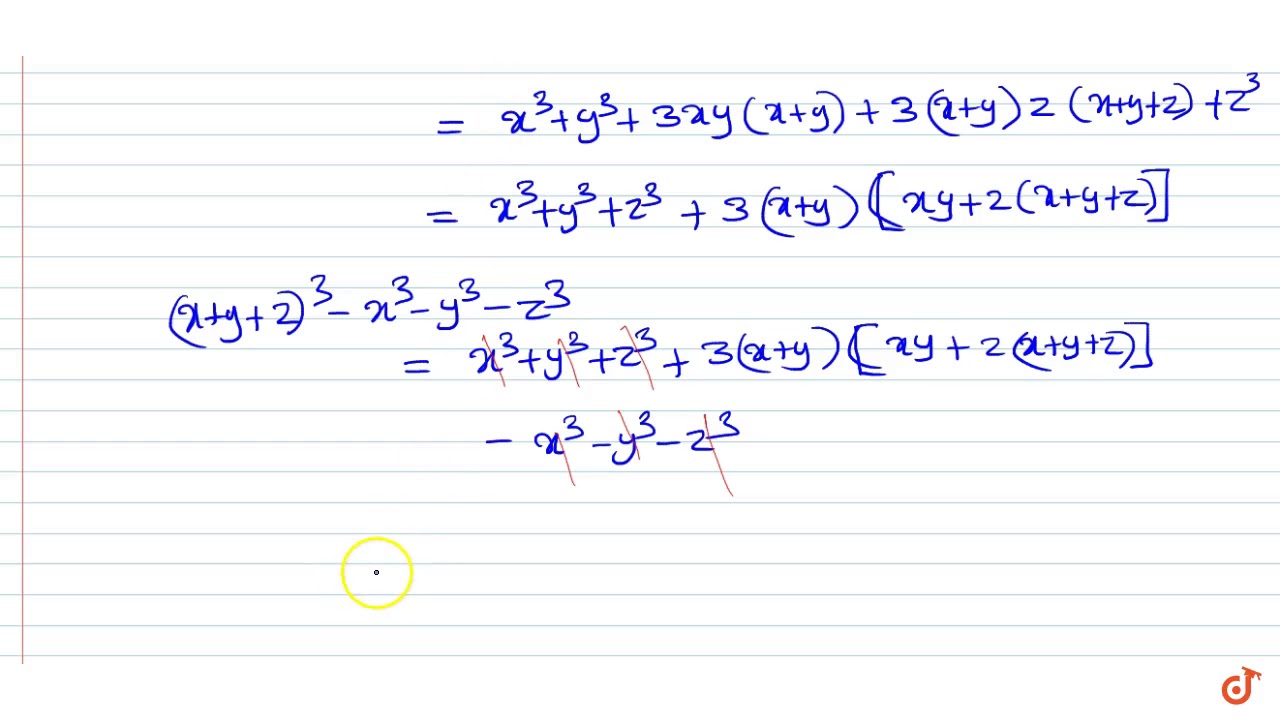



Factorise The Expression X Y Z 3 X 3 Y 3 Z 3 Into Linear Factors Youtube
Find an answer to your question factorise x^33x^2y3xy^2y^38 1007 1007 Math Secondary School answered Factorise x^33x^2y3xy^2y^38 2 See answers BrainlyHulk BrainlyHulk factorise x x y 3 3x2y x Mathematics TopperLearningcom Starting early can help you score better!#x^3 3x^2y 3xy^2 y^3 #64 #x^3 3x^2y 3xy^2 y^3 # 64 Algebra 1 Answer Narad T Please see the explanation below Explanation We need #a^3b^3=(ab)(a^2abb^2)# #(ab)^3=a^33a^2b3ab^2b^3#




Consider The Equation X 2y 3 X 1 Chegg Com




Factoring Factorization Arithmetic
Order my "Ultimate Formulmznto/2ZDeifD Hire me for private lessons https//wyzantcom/tutors/jjthetutorRead "The 7 Habits of Successful STHola a todos, en este videos vamos a resolver esta derivada por el método de la regla de la cadena para funciones de varias variables, este es el caso 2 porq3xy=5;x2y=3 Simple and best practice solution for 3xy=5;x2y=3 Check how easy it is, to solve this system of equations and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework




Factorise X X Y 2 3x2y X Y Maths Polynomials Meritnation Com



How To Factor X 3 X 2 Xy X Y 1 Quora
How do you factor #x^38y^3#?Factorise (3x 2y)2 3 (3x 2y) 10 CISCE ICSE Class 9 Question Papers 10 Textbook Solutions Important Solutions 5 Question Bank Solutions Concept Notes & Videos 258 Syllabus Advertisement Remove all adsSolve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more




Math Problem Factorization X 4y X 2y 3 X 3y 2 Xy 4 Youtube




Factorize X X Y 2 3x2y X Y Brainly In




Ex 9 4 3 V Simplify X Y 2x Y X 2y X Y Class 8




Factors Of X 3 X 2y Xy 2 Y 3 Brainly In




Differential Equations Solved Examples Show That Following Differential Equation Is Not Exact 3x 2y 4 2xy Dx 2x 3y 3 X 2 Dy 0 Then Find An Integrating Factor To Solve The Differential Equation




3x Y Y 2 Dx X 2 X Y Dy 0 Youtube




How To Factorise A Polynomial By Splitting The Middle Term A Plus Topper
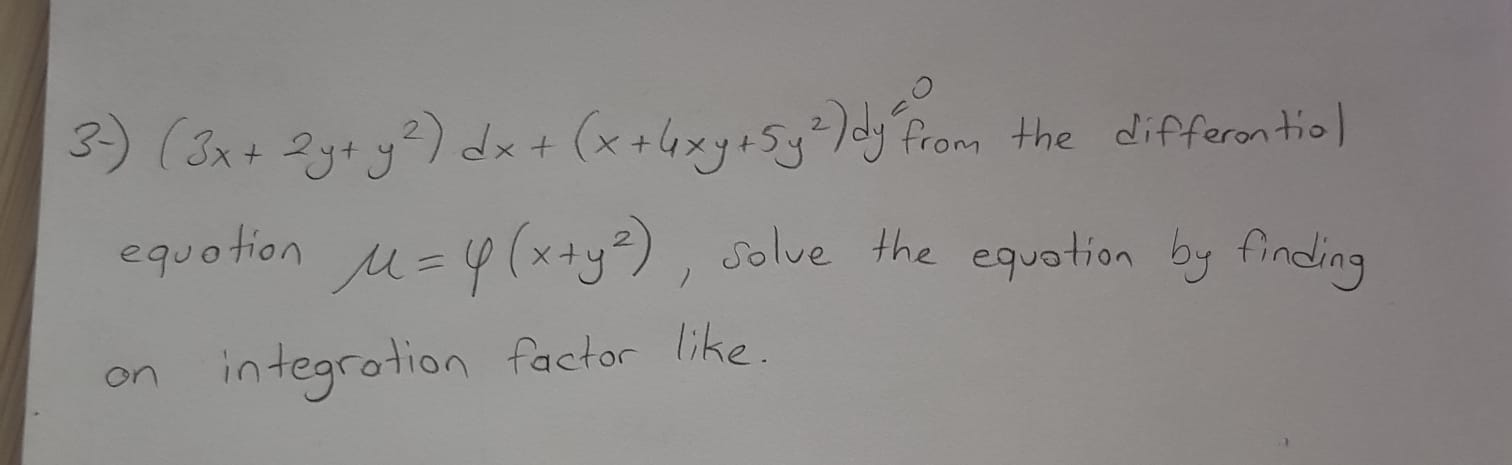



Solved 3 3x 2 Y Y Dx X 4 Xy 5 Y Dy From Chegg Com



Q Tbn And9gcqcxcgn0u5z5eyedtk36btsho Ukuwl31vgadu0c58hdwsxafpg Usqp Cau



If X Y 3 X Y 1 Then What Is X Y Quora




Ex 14 1 3 I Factorise X 2 X Y 8x 8y Ex 14 1



What Is The Solution Of The Differential Equation Math Xy 3 Y Dx 2 X 2y 2 X Y 4 Dy 0 Math Quora




Chapter 6 Review Factor The Expression 1 6x 3 2 X3 4x2 3 4z




Factorise The Following Expression X X Y 2 3x 2y X Y Brainly In
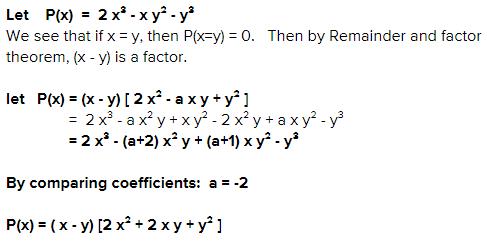



Factorise 2x 3 Xy 2 Y 3 Edurev Class 9 Question




Choose The Best Method And Factor The Following Chegg Com



How To Solve The Following Equation In Minimum Time If 8 X Y 3 X Y 3 X 3y Ax 2 Bxy Cy 2 Then The Value Of A B And C Is Quora




Solve The Following Using Separation Of Variables A Chegg Com
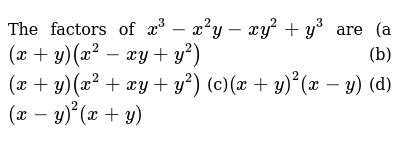



The Factors Of X 3 X 2y X Y 2 Y 3 Are A X Y X 2 X Y Y 2




How To Know At A Glance That Math X 3 3xy 2 2y 3 Math Is Factorable Quora




A Show That The Differential Equation X 2y 3 X Chegg Com
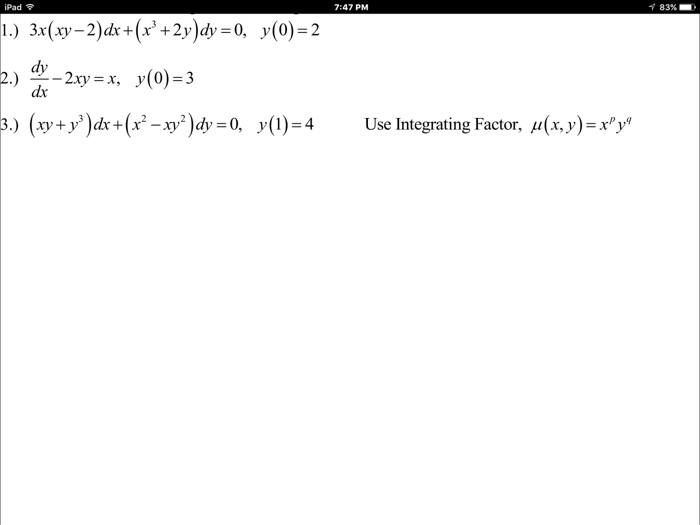



3x Xy 2 Dx X 3 2y Dy 0 Y 0 2 Dy Dx Chegg Com
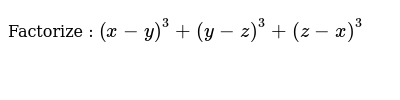



Factorize X X Y 3 3x 2y X Y




Factorise X 3 3x 2y 3xy 2 2y 3 Brainly In



How To Solve X 3 3xy 2 Dx 3x 2y Y 3 Dy Quora




Expand X X Y 3 3xy X Y




Factorise X X 3 3 3x 2y X Y Brainly In



If X Y 3 X Y 1 Then What Is X Y Quora




X X Y 3 3x 2y X Y Factorise This Brainly In




Factorize X Xy 3 3x 2y Xy Youtube




Math 432 Hw 2 5 Solutions Frostburg




Factorise X X Y 3 3x 2y X Y Brainly In



Factorise X X Y 3 3x2y X Mathematics Topperlearning Com




Factorizing If 2x 1 Is A Factor Of The Expression 6 X2 5x



Search Q Factorise 6ab B 5e2 2b12ac 2bc Tbm Isch




To Solve X Y 3 3x 2y 4 0 By Determinant Method Find D Brainly In
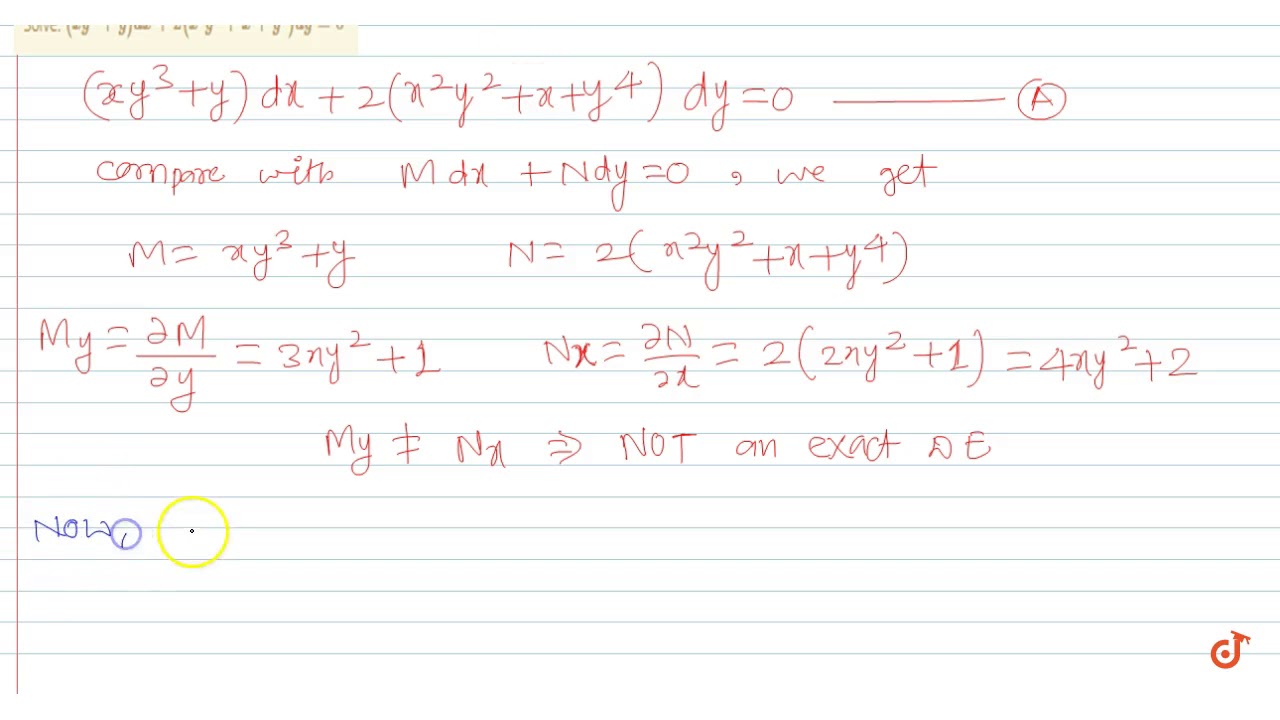



Solve Xy 3 Y Dx 2 X 2y 2 X Y 4 Dy 0 Youtube



How To Factorise 3 X Y 2 2 X Y Quora
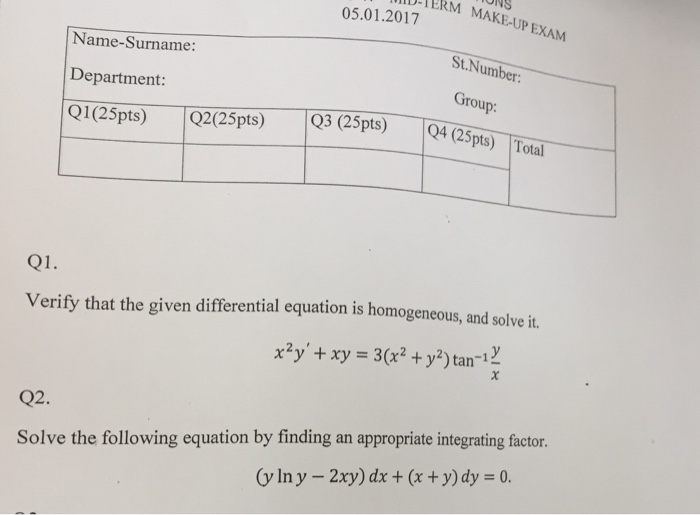



Solved Verify That The Given Differential Equation Is Hom Chegg Com
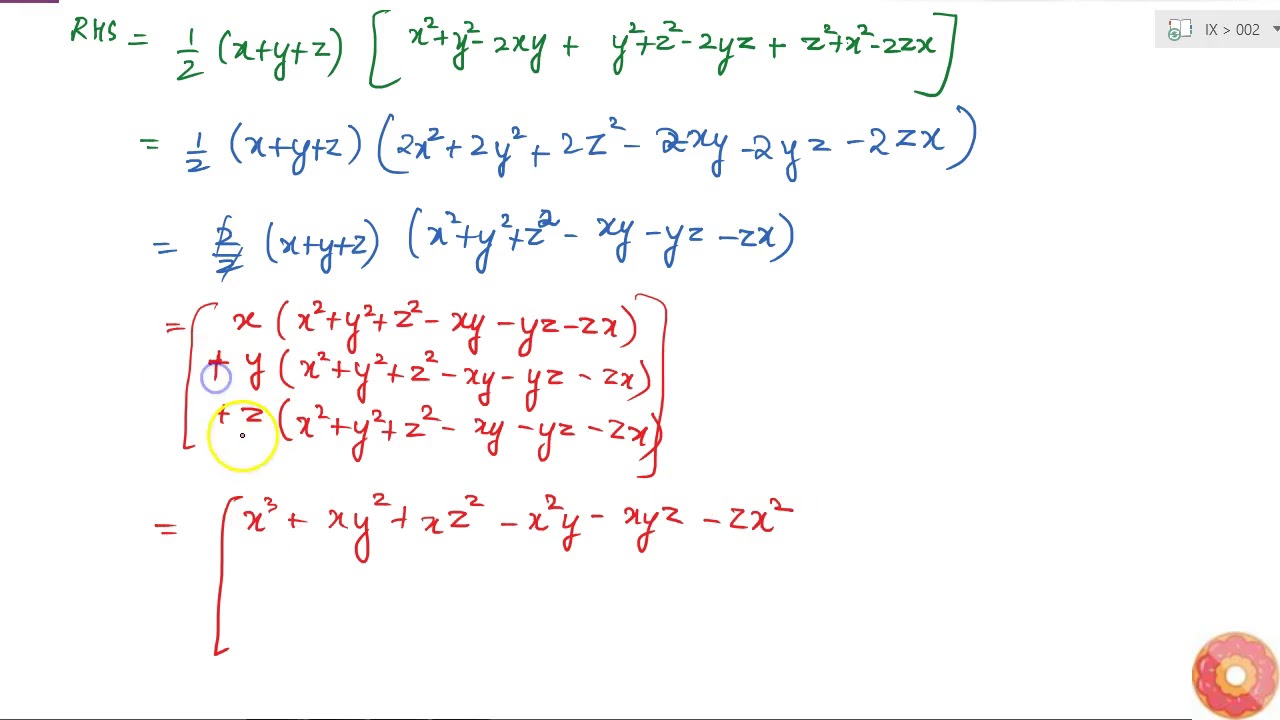



Verify That X 3 Y 3 Z 3 3x Y Z 1 2 X Y Z X Y 2 Y Z 2 Z X 2 Youtube




The Factors Of X 3 1 Y 3 3x Y Are A X 1 Y X 2 1 Y 2 X Y



What Is The Solution For Math Xy 2 2x 2y 3 Dx X 2y X 3y 2 Dy 0 Math Quora




Chapter 3 More About Factorization Ppt Download




Find The Special Integrating Factor To Make The Given Chegg Com




Differential Equation 1st Order Reducible To Separable Forms 5 Of 7 Example 4 X 2y X 2 Xy Y 2 Youtube




X 3 3xy 2 Dx Y 3 3x 2y Dy Youtube
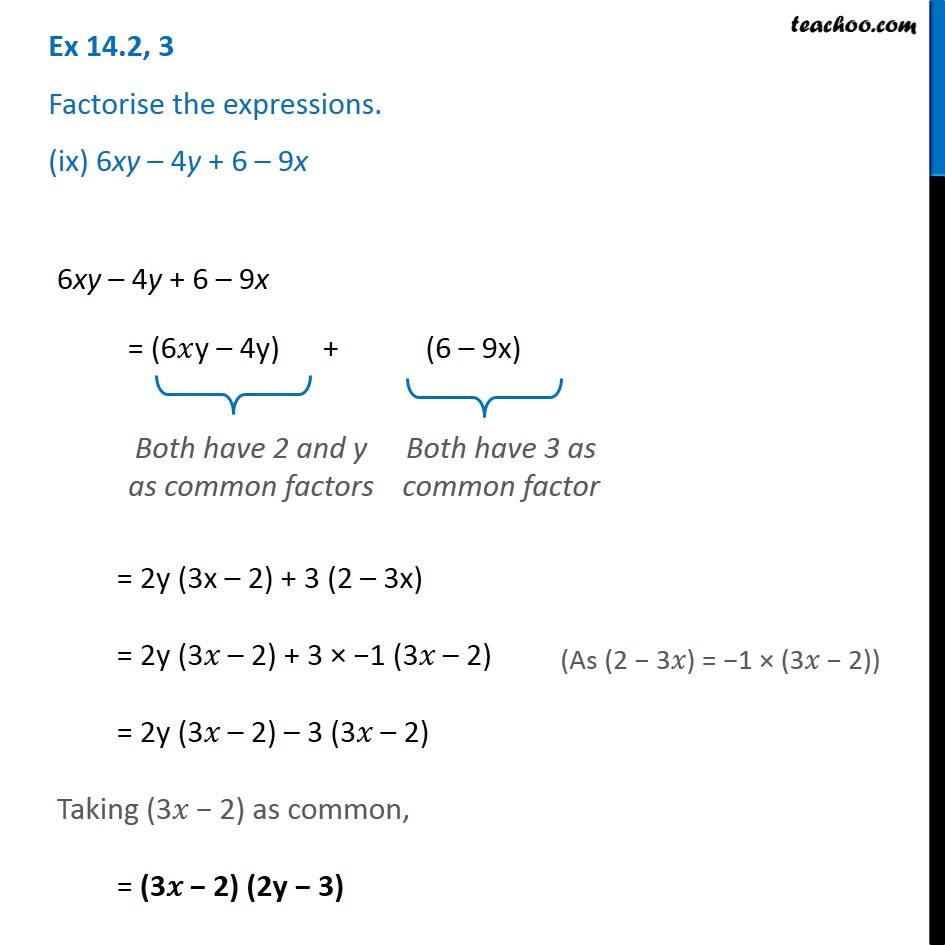



Ex 14 2 3 Ix Factorise 6xy 4y 6 9x Chapter 14 Ncert




Solutions To Implicit Differentiation Problems



If Math X Y 3 Math And Math X 2 Y 2 3 Math Then What Is The Value Of Math X 2 Y 2 Math Quora




Factorize X X 3 Y 3 3x Y X Y
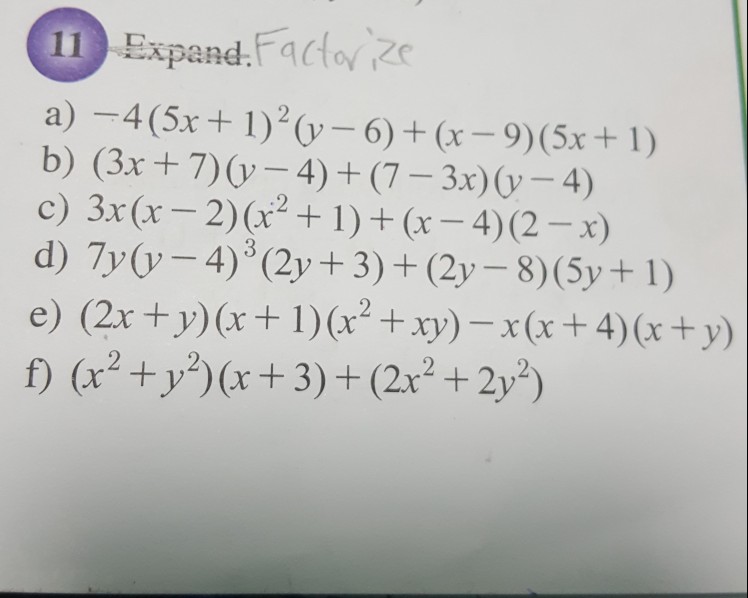



A 4 5x 1 2 Y 6 R 9 5x 1 B 3x 7 4 Chegg Com
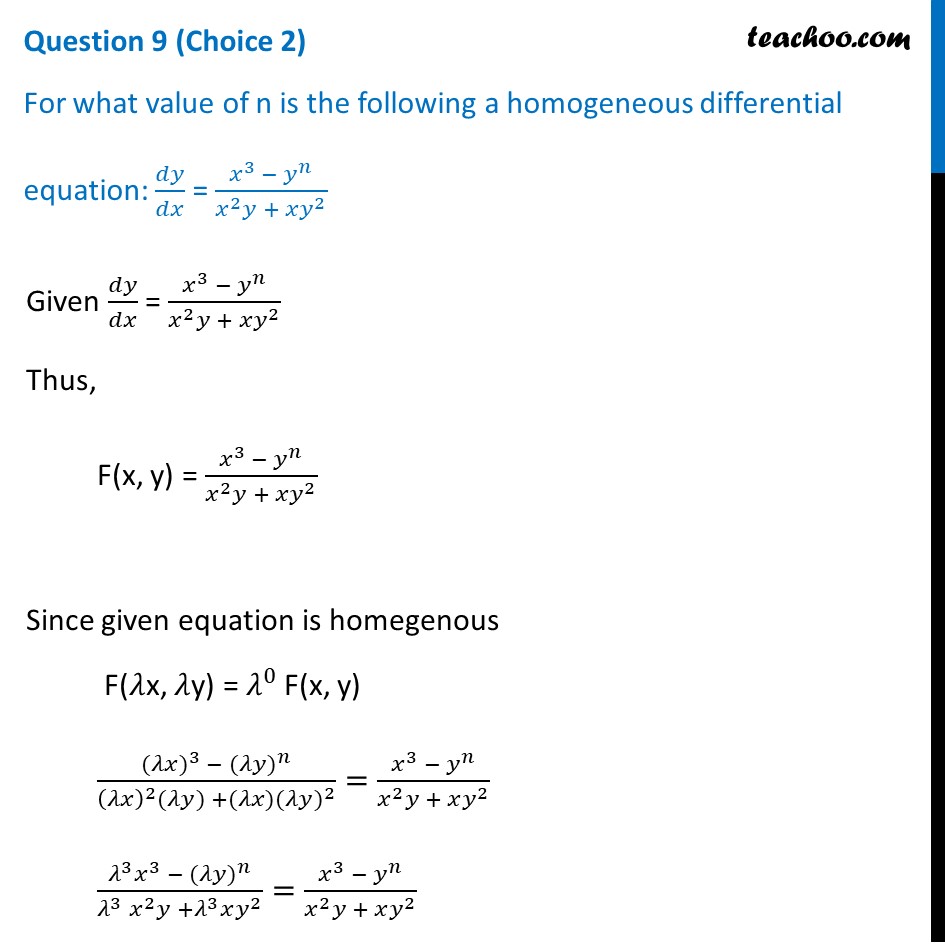



For What Value Of N Is Following A Homogeneous Differential Equation




The Factors Of X 3 X 2y X Y 2 Y 3 Are A X Y X 2 X Y Y 2



Factorize X X Y 3 3x 2y X Y




Factorize 8 X Y 3 27 X Y 3 Youtube



How To Solve Math Xy 2 Dy X 3 Y 3 Dx 0 Math Quora




Factorise Completely X 2y 3x Xy 3 Brainly In




Simplify The Following X 2y Y 3x X Y X 3y Y 3x 4x 5y



How To Solve The General Solution Of X 3 Y 3 Dx Xy 2dy 0 Quora




Factorize 2xy 3 2y 3x Maths Questions




Find The Asymptotes Of The Following Curves Y 3 3x 2y X Y 2 3x 3 2y 2 2x Y 4x 5y 6 0 Youtube




X 2 2y 3 1 X Y 3 3 Solve The Given Equation Using Elimination And Substitution Method Youtube
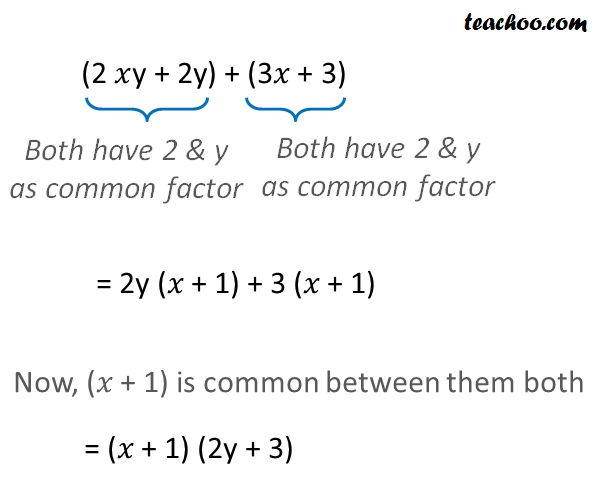



Factorisation Of Algebra By Regrouping Terms Teachoo Factorisation




Solve This X X Y 3 3x 2y X Y Mathematics Topperlearning Com Ynhf0tnn
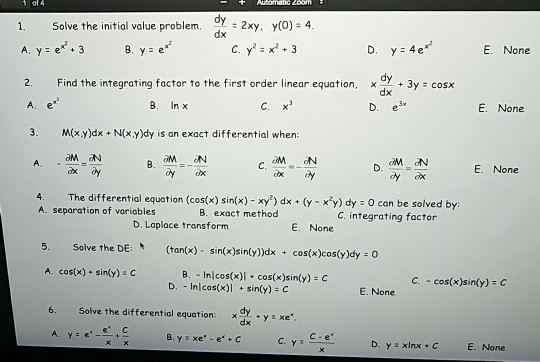



Solve The Initial Value Problem Dy Dx 2 Xy Y 0 Chegg Com




Math 432 Hw 2 5 Solutions




Factorize X X Y 3 3x 2y X Y Brainly In




Solve The Equation 3x2 3x 2y Dx X2y X 1 Dy0 An Chegg Com




Simplify 64 X X Y 3 2 Express In Scientific Chegg Com




What Are The Factors Of X Y 3 X3 Y3 Brainly In
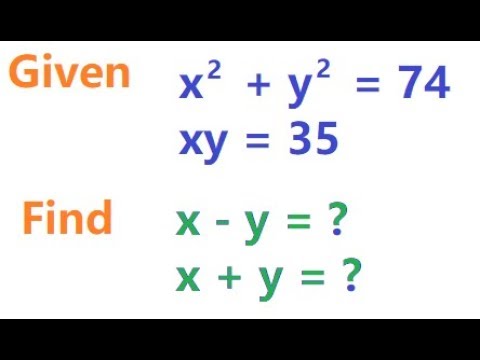



X 2 Y 2 74 And Xy 35 Find X Y And X Y 2x 3y 14 And Xy 8 Find 4x 2 9y 2 Youtube




Factor X X Y 3 3x 2y X Y Brainly In




X 3 3x 2 X 3 Factorise Novocom Top




Differential Equations Solved Examples Show That Following Differential Equation Is Not Exact 3x 2y 4 2xy Dx 2x 3y 3 X 2 Dy 0 Then Find An Integrating Factor To Solve The Differential Equation




Mathematics 1 3 Factor Fully A 9x 18x 6x B Chegg Com




Rd Sharma Class 9 Chapter 5 Factorization Of Algebraic Expressions




The Solution Of The Differential Equation 1 Y X 2y Dx X



1




Factor X X Y 3 3x 2y X Y Brainly In




Solve The Following Differential Equations 1 3x 2y 4 2xy Dx 2x 3y 3 X 2 Dy 0 Youtube



No comments:
Post a Comment